FE EXAM PREP – HOW TO SOLVE ENGINEERING PROBLEMS
This post will attempt to provide a repetitive process that we can use to solve any FE type – engineering problem that we encounter as we prepare for the FE exam. These steps will be specific to the FE exam, but many engineering students or professionals may find the techniques used helpful. We will go through a step by step process to solve a calculation-based Mechanics of Materials FE type question.
Materials Needed
For the FE exam we are only allowed to use:
- Pencil and Writing Pad
- FE Reference Handbook 10.0.1 (PDF Version) (https://account.ncees.org/reference-handbooks/)
- NCEES approved calculator (https://ncees.org/exams/calculator/)

Since most of the necessary equations needed to solve FE exam questions are provided in the FE Reference Handbook, we will heavily refer to this as run through the step by step process to solve a sample problem.
Now, let’s dive right into it.
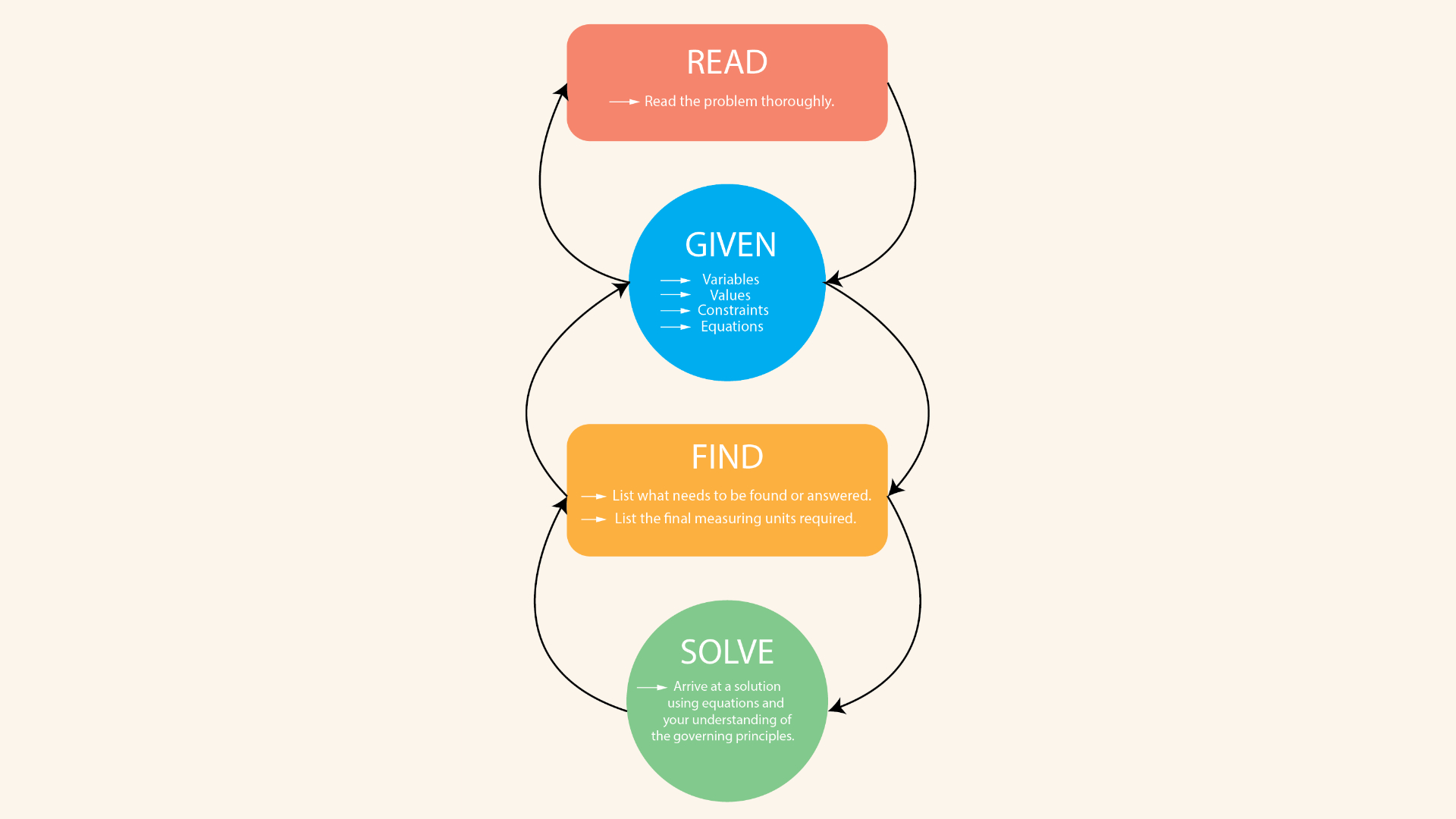
We will,
READ the problem statement thoroughly, extract everything we are GIVEN, state what we need to FIND, and SOLVE.

Here is our example problem statement.
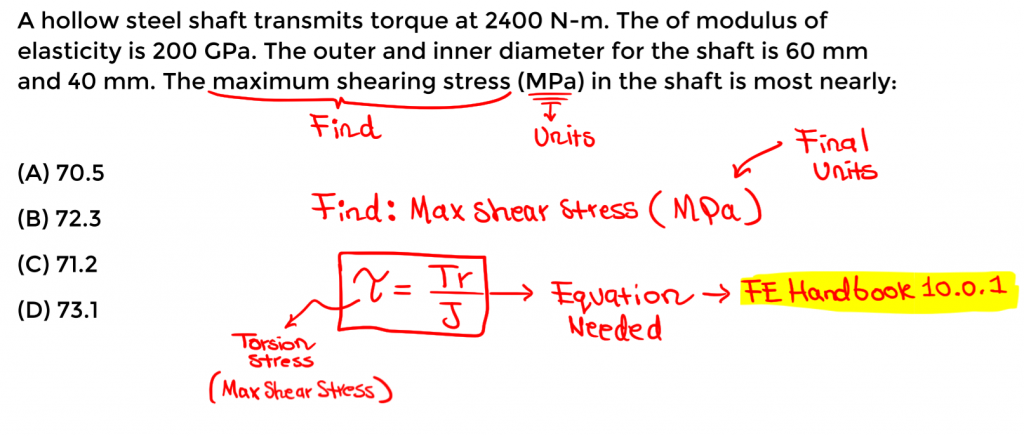
Problem Statement
A hollow steel shaft transmits torque at 2400 N-m. The modulus of elasticity is 200 GPa. The outer and inner diameter for the shaft is 60 mm and 40 mm. The maximum shearing stress (MPa) in the shaft is most nearly:
(A) 70.5
(B) 72.3
(C) 71.2
(D) 73.1
STEP 1
READ
This is a vital step in the overall process. Even though we are under a time constraint, we cannot simply scan through the problem since we might miss important information needed to solve the problem. Sometimes figures and tables will be provided to us in the problem statement, therefore, as we read the problem, we must try to relate the sentences to these figures/tables and vice versa. The goal is to read the problem with a PURPOSE answer the following questions:
- What information is GIVEN in the problem statement?
- What do will I need to FIND?
- Do I have everything I need to SOLVE the problem?
STEP 2
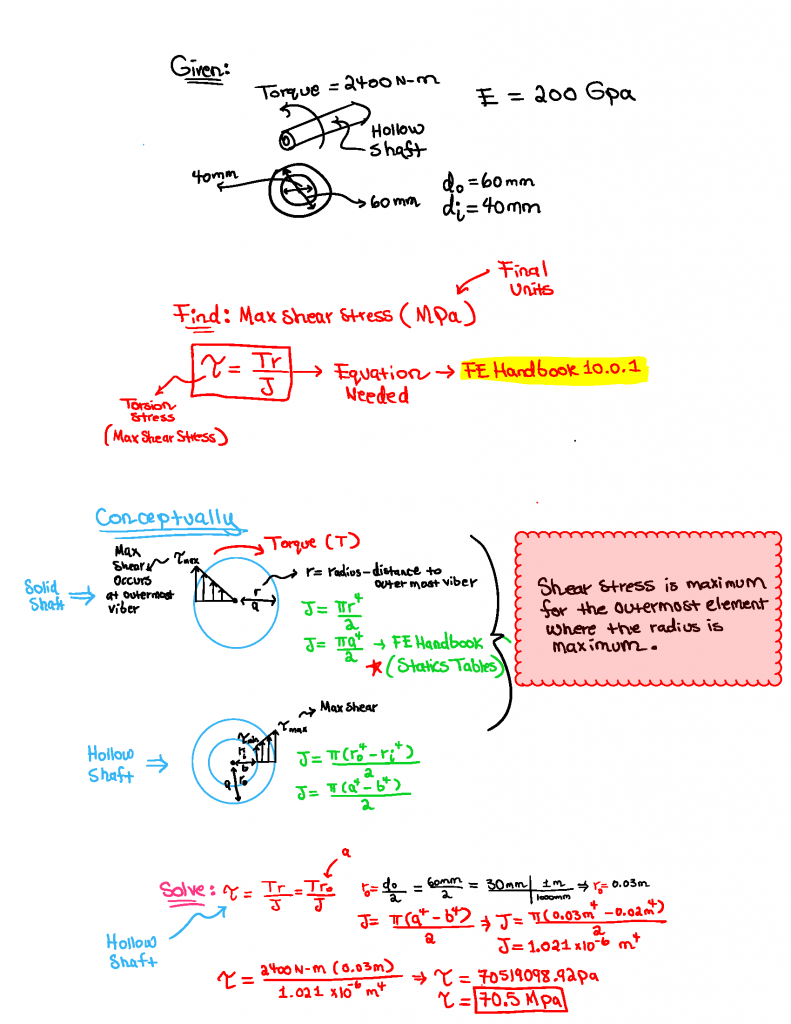
GIVEN
As we read the problem statement, we must extract all information provided. We will simultaneously do two things:
- We will begin visualizing the problem by rough sketching the physical objects involve or drawing a free body diagram if needed.
- We will list everything that is given including constraints, values, and variables.
We do not have to have the most accurate sketch. The goal is to visualize the basic shapes involved and to try to assign each numerical value provided to an engineering variable (σ,τ,η, E, etc, etc).
Here is an example of what you might get for the GIVEN.

STEP 3
FIND
After taking a mental and visual note of everything we are given; we will apply the following steps:
- List what we need to find.
- List the final units required for our final answer.
Listing what we need to FIND and Listing the UNITS needed.
We must make sure that we understand what is being asked. ALL FE exam problems will directly ask us to find or identify something. For calculation-based questions, the final units needed will also be listed in the problem statement. Here is an example of what you might get:

STEP 4
SOLVE
Now, all we have to do is to use an equation and solve right? Unfortunately, no. It won’t be that simple.
To apply any equation we use, we MUST UNDERSTAND THE CONCEPT.
Before we solve. We will have to:
Write down the relevant equation(s) and know how to apply the equation.
Some FE questions will require us to use an equation or multiple equations to arrive at a final answer. Sometimes it will be simple, as it is in this sample problem, but at times we will be required to have a basic understanding of the concept to know what equation to use. We can develop this conceptual understanding only through plenty of practice and the desire to understand the governing principles (Bernoulli, continuity, equilibrium, etc.) that may apply to each problem we go through.
The crucial step here is to get used to searching through the FE Reference Handbook using (CTRL-F, CMD-F). For this specific question, we will search for the keyword “shaft” – since this is the “subject” or object that is being dealt with. As shown below, this directs us to a torsion stress equation (also known as the maximum shear stress).
From the equation, we know here that the torsion stress will depend on the torque (T), the radius to the outermost fiber (r), and the polar moment of inertia (J).
ASSUMING WE HAVE NEVER SOLVED TORSION STRESS PROBLEMS BEFORE, THE ONLY WAY WE CAN SOLVE THIS QUESTION IS BY CONCEPTUALLY UNDERSTANDING THE ROLE EACH VARIABLE PLAYS IN THIS EQUATION SINCE THE VARIABLES (r) AND (J) ARE NOT CLEARLY DEFINED ON THAT PAGE IN THE FE REFERENCE HANDBOOK.
This is where we must take the initiative to conceptually understand how to apply the equation. We can begin to take notes covering the topic of torsional stress in several ways:
- Googling an article discussing torsion stress.
- Googling images discussing torsion stress.
- Watching a short YouTube video covering torsion stress.
- Watching a portion of an online course covering torsion stress.
- Reading an article or a section of a text covering torsion stress.
- Reading a specific FE exam book that conceptually covers the topic of torsion stress.
After doing a quick google search for articles and images, we can gather ALL the relevant conceptual information we need to solve this question.
Here is an example of how your notes might look like.
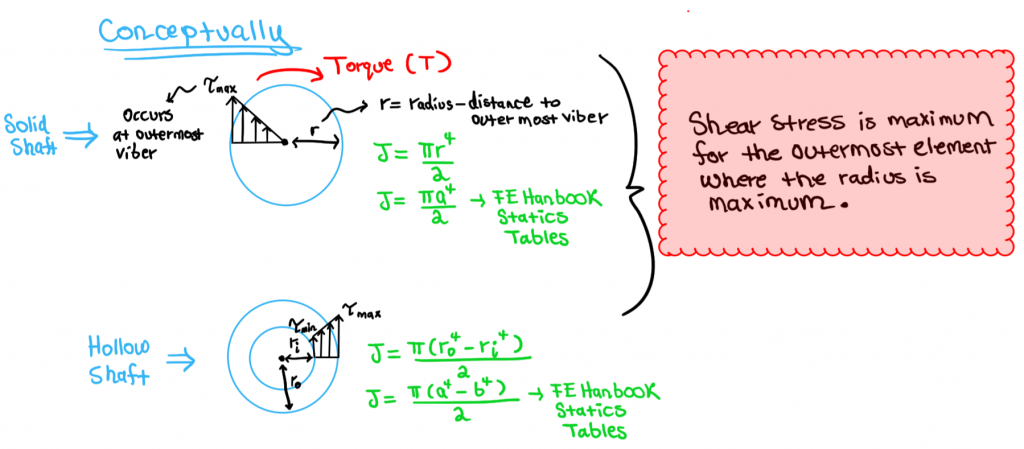
With all the given information listed and a robust understanding of how to use the torsion stress equation, we can finally SOLVE the problem as shown by the figure below.

Notice how if we did not conceptually understand how to use the torsion stress equation for a hollow shaft, we would have most likely solved this question incorrectly. Therefore, as you prepare for your FE exam, you must begin to understand all the variables involved in each equation you intend to use.
FINAL SHEET